The Carol numbers up to 1015 :
7, 47, 223, 959, 3967, 16127, 65023, 261119, 1046527, 4190207, 16769023, 67092479, 268402687, 1073676287, 4294836223, 17179607039, 68718952447, 274876858367, 1099509530623, 4398042316799, 17592177655807, 70368727400447, 281474943156223.
Distribution of the remainders when the numbers in this family are divided by n=2, 3,..., 11. (I took into account 100000 values, from 7 to 3.992⋅1060206).
| n\r | 0 | 1 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 0 | 100000 | 2 | ||||||||
| 3 | 0 | 50000 | 50000 | 3 | |||||||
| 4 | 0 | 0 | 0 | 100000 | 4 | ||||||
| 5 | 0 | 0 | 50000 | 25000 | 25000 | 5 | |||||
| 6 | 0 | 50000 | 0 | 0 | 0 | 50000 | 6 | ||||
| 7 | 33334 | 0 | 0 | 0 | 0 | 33333 | 33333 | 7 | |||
| 8 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 100000 | 8 | ||
| 9 | 0 | 0 | 16667 | 0 | 0 | 16667 | 0 | 50000 | 16666 | 9 | |
| 10 | 0 | 0 | 0 | 25000 | 0 | 0 | 0 | 50000 | 0 | 25000 | 10 |
| 11 | 0 | 20000 | 20000 | 20000 | 0 | 0 | 0 | 20000 | 0 | 10000 | 10000 |
A pictorial representation of the table above
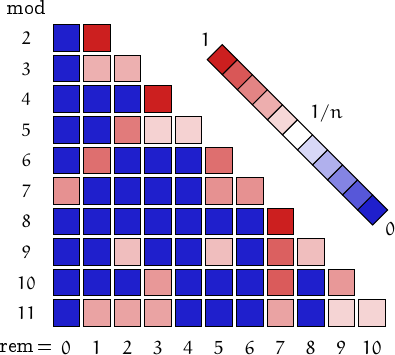
Imagine to divide the members of this family by a number n and compute the remainders. Should they be uniformly distributed, each remainder from 0 to n-1 would be obtained in about (1/n)-th of the cases. This outcome is represented by a white square. Reddish (resp. bluish) squares represent remainders which appear more (resp. less) frequently than 1/n.
e-mail: info -at- numbersaplenty.com • Privacy notice • engine limits