The narcissistic numbers up to 1015 :
1, 2, 3, 4, 5, 6, 7, 8, 9, 153, 370, 371, 407, 1634, 8208, 9474, 54748, 92727, 93084, 548834, 1741725, 4210818, 9800817, 9926315, 24678050, 24678051, 88593477, 146511208, 472335975, 534494836, 912985153, 4679307774, 32164049650, 32164049651, 40028394225, 42678290603, 44708635679, 49388550606, 82693916578, 94204591914, 28116440335967.
Distribution of the remainders when the numbers in this family are divided by n=2, 3,..., 11. (I took into account 88 values, from 1 to 1.15⋅1038).
| n\r | 0 | 1 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 44 | 44 | 2 | ||||||||
| 3 | 44 | 20 | 24 | 3 | |||||||
| 4 | 17 | 18 | 27 | 26 | 4 | ||||||
| 5 | 20 | 17 | 17 | 17 | 17 | 5 | |||||
| 6 | 21 | 8 | 11 | 23 | 12 | 13 | 6 | ||||
| 7 | 11 | 17 | 8 | 13 | 13 | 10 | 16 | 7 | |||
| 8 | 7 | 9 | 19 | 14 | 10 | 9 | 8 | 12 | 8 | ||
| 9 | 18 | 10 | 7 | 13 | 5 | 11 | 13 | 5 | 6 | 9 | |
| 10 | 8 | 9 | 8 | 7 | 10 | 12 | 8 | 9 | 10 | 7 | 10 |
| 11 | 7 | 7 | 5 | 8 | 6 | 6 | 10 | 12 | 10 | 7 | 10 |
A pictorial representation of the table above
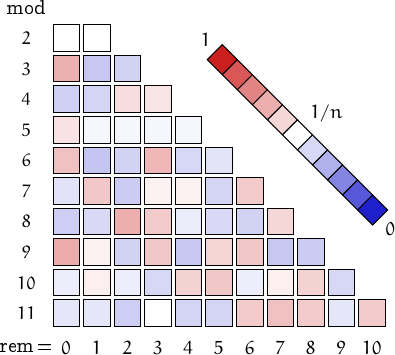
Imagine to divide the members of this family by a number n and compute the remainders. Should they be uniformly distributed, each remainder from 0 to n-1 would be obtained in about (1/n)-th of the cases. This outcome is represented by a white square. Reddish (resp. bluish) squares represent remainders which appear more (resp. less) frequently than 1/n.
e-mail: info -at- numbersaplenty.com • Privacy notice • engine limits