• 2 can be written using four 4's:
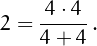
2 has 2 divisors, whose sum is σ = 3. Its totient is φ = 1.
The next prime is 3.
It is the 3-rd Fibonacci number F3.
It is a primorial, being the product of the first 1 primes.
It is a factorial (2 = 2 ! = 1 ⋅ 2 ), and thus also a Jordan-Polya number.
It is a double factorial (2 = 2 !! = 2 ).
2 is digitally balanced in base 2, because in such base it contains all the possibile digits an equal number of times.
2 is an esthetic number in base 2, because in such base its adjacent digits differ by 1.
It can be written as a sum of positive squares in only one way, i.e., 1 + 1 = 1^2 + 1^2 .
2 is a sliding number.
It is a tau number, because it is divible by the number of its divisors (2).
It is the 2-nd Motzkin number.
Together with 1093 it forms a Wieferich pair.
It is a cyclic number.
It is the 2-nd Bell number.
It is the 2-nd Catalan number.
It is a Sophie Germain prime.
It is a Chen prime.
It is a Harshad number since it is a multiple of its sum of digits (2).
It is a super Niven number, because it is divisible the sum of any subset of its (nonzero) digits.
It is a nude number because it is divisible by every one of its digits and also a Zuckerman number because it is divisible by the product of its digits.
It is an iccanobiF number.
2 is an idoneal number.
It is a tribonacci number.
It is a tetranacci number.
It is an Ulam number.
It is (trivially) a d-powerful number and an alternating number.
It is a cake number, because a cake can be divided into 2 parts by 1 planar cuts.
It is a pancake number, because a pancake can be divided into 2 parts by 1 straight cuts.
It is a Kynea number, being equal to (20 + 1)2 - 2.
It is one of the 548 Lynch-Bell numbers.
2 is strictly pandigital in base 2.
It is the 2-nd Perrin number.
It is a Curzon number.
It is the 2-nd primeval number, because it sets a new record (1) in the number of distinct primes that is it possible to write using its digits.
It is a partition number, being equal to the number of ways a set of 2 identical objects can be partitioned into subset.
It is a nialpdrome in base 2.
It is a self number, because there is not a number n which added to its sum of digits gives 2.
It is a panconsummate number.
2 is an untouchable number, because it is not equal to the sum of proper divisors of any number.
It is a subfactorial, being equal to the number of derangements of 3 objects .
It is a good prime.
It is a Pierpont prime, being equal to 20 ⋅ 30 + 1.
It is an impolite number, since it cannot be written as a nontrivial sum of consecutive naturals.
It is a (trivial) narcissistic number.
2 is a highly composite number, because it has more divisors than any smaller number.
2 is a superabundant number, because it has a larger abundancy index than any smaller number.
It is a pronic number, being equal to 1×2.
It is a practical number, because each smaller number is the sum of distinct divisors of 2
2 is a deficient number, since it is larger than the sum of its proper divisors (1).
2 is an equidigital number, since it uses as much as digits as its factorization.
With its successor (3) it forms an eRAP, since the sums of their prime factors are consecutive (2 and 3).
2 is an odious number, because the sum of its binary digits is odd.
The product of its digits is 2, while the sum is 2.
The square root of 2 is about 1.4142135624. The cubic root of 2 is about 1.2599210499.
The spelling of 2 in words is "two", and thus it is an aban number, an eban number, an iban number, and an uban number.
• e-mail: info -at- numbersaplenty.com • Privacy notice • done in 0.878 sec. • engine limits •

