• 726 can be written using four 4's:

See also 113.

---

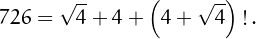
726 has 12 divisors (see below), whose sum is σ = 1596. Its totient is φ = 220.

The previous prime is 719. The next prime is 727. The reversal of 726 is 627.

726 is nontrivially palindromic in base 5.

726 is an esthetic number in base 6, because in such base its adjacent digits differ by 1.

It is a Curzon number.

It is a plaindrome in base 14 and base 15.

It is a nialpdrome in base 3, base 6, base 9 and base 11.

It is a congruent number.

It is not an unprimeable number, because it can be changed into a prime (727) by changing a digit.

726 is an untouchable number, because it is not equal to the sum of proper divisors of any number.

It is a polite number, since it can be written in 5 ways as a sum of consecutive naturals, for example, 61 + ... + 71.

It is an arithmetic number, because the mean of its divisors is an integer number (133).

It is a practical number, because each smaller number is the sum of distinct divisors of 726, and also a Zumkeller number, because its divisors can be partitioned in two sets with the same sum (798).

726 is an abundant number, since it is smaller than the sum of its proper divisors (870).

It is a pseudoperfect number, because it is the sum of a subset of its proper divisors.

726 is a wasteful number, since it uses less digits than its factorization.

726 is an evil number, because the sum of its binary digits is even.

The sum of its prime factors is 27 (or 16 counting only the distinct ones).

The product of its digits is 84, while the sum is 15.

The square root of 726 is about 26.9443871706. The cubic root of 726 is about 8.9876373471.

Adding to 726 its sum of digits (15), we get a triangular number (741 = T₃₈).

Subtracting from 726 its reverse (627), we obtain a palindrome (99).

It can be divided in two parts, 72 and 6, that added together give a triangular number (78 = T₁₂).

The spelling of 726 in words is "seven hundred twenty-six", and thus it is an aban number and an oban number.