The panconsummate numbers up to 106 :
1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 14, 15, 18, 20, 21, 23, 24, 31, 34, 36, 37, 39, 40, 43, 45, 53, 54, 57, 59, 61, 69, 72, 73, 77, 78, 81, 85, 89, 91, 121, 127, 144, 166, 169, 211, 219, 231, 239, 257, 267, 271, 331, 337, 353, 361, 413, 481, 523, 571, 661, 721, 1093, 1291, 3097.
Distribution of the remainders when the numbers in this family are divided by n=2, 3,..., 11. (I took into account 66 values, from 1 to 3097).
| n\r | 0 | 1 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 18 | 48 | 2 | ||||||||
| 3 | 21 | 30 | 15 | 3 | |||||||
| 4 | 9 | 27 | 9 | 21 | 4 | ||||||
| 5 | 7 | 21 | 12 | 12 | 14 | 5 | |||||
| 6 | 9 | 25 | 4 | 12 | 5 | 11 | 6 | ||||
| 7 | 8 | 15 | 8 | 11 | 9 | 11 | 4 | 7 | |||
| 8 | 5 | 15 | 4 | 12 | 4 | 12 | 5 | 9 | 8 | ||
| 9 | 8 | 11 | 4 | 6 | 13 | 7 | 7 | 6 | 4 | 9 | |
| 10 | 3 | 18 | 3 | 9 | 6 | 4 | 3 | 9 | 3 | 8 | 10 |
| 11 | 4 | 12 | 4 | 6 | 9 | 1 | 9 | 6 | 4 | 5 | 6 |
A pictorial representation of the table above
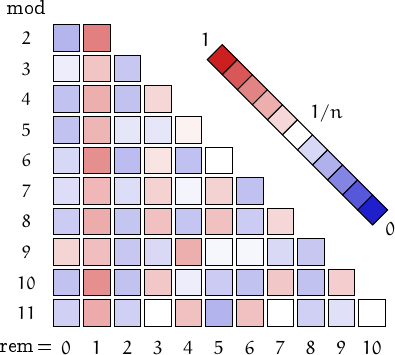
Imagine to divide the members of this family by a number n and compute the remainders. Should they be uniformly distributed, each remainder from 0 to n-1 would be obtained in about (1/n)-th of the cases. This outcome is represented by a white square. Reddish (resp. bluish) squares represent remainders which appear more (resp. less) frequently than 1/n.
e-mail: info -at- numbersaplenty.com • Privacy notice • engine limits