A number 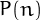 is as partition number if it is equal to the number of ways in which a set of
is as partition number if it is equal to the number of ways in which a set of 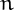 identical objects can be partitioned.
identical objects can be partitioned.
![\[
P(n) =\frac{1}{n}\sum_{k=0}^{n-1}\sigma(n-k)P(k)\,.
\]](pic.9.png)
![\[ P(n) \approx \frac{1}{4n\sqrt{3}} e^{\pi\sqrt{2n/3}}\,.\]](pic.10.png)
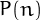 is as partition number if it is equal to the number of ways in which a set of
is as partition number if it is equal to the number of ways in which a set of 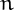 identical objects can be partitioned.
identical objects can be partitioned.
For example, 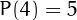 because 4 objects can be partitioned in 5 ways (1)
because 4 objects can be partitioned in 5 ways (1) 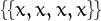 , (2)
, (2) 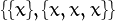 , (3)
, (3) 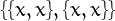 , (4)
, (4)  , and (5)
, and (5) 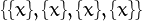 .
.
There are many recursive formulas for 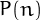 . For example,
. For example,
![\[
P(n) =\frac{1}{n}\sum_{k=0}^{n-1}\sigma(n-k)P(k)\,.
\]](pic.9.png)
Hardy and Ramanujan (1918) obtained the asymptotic approximation
![\[ P(n) \approx \frac{1}{4n\sqrt{3}} e^{\pi\sqrt{2n/3}}\,.\]](pic.10.png)
The first partition numbers are 1, 2, 3, 5, 7, 11, 15, 22, 30, 42, 56, 77, 101, 135, 176, 231, 297, 385, 490, 627, 792, 1002, 1255, 1575, 1958, 2436, 3010, 3718, 4565, 5604, 6842, 8349, 10143 more terms
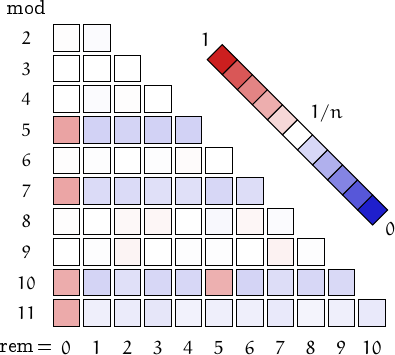
Pictorial representation of remainders (mod 2, 3, ...,11) frequency. For a table of values and more details click here

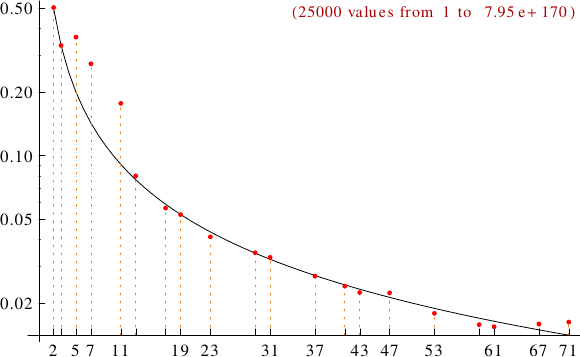
A graph displaying how many partition numbers are multiples of the primes p from 2 to 71. In black the ideal line 1/p.

Useful links
Mathworld, Partition fuction P
Wikipedia, Partition (number theory)
OEIS, Sequence A000041
Wikipedia, Partition (number theory)
OEIS, Sequence A000041
Partition numbers can also be... (you may click on names or numbers and on + to get more values)
a-pointer
11
101
aban
11
15
+
627
792
abundant
30
42
+
15796476
26543660
admirable
30
42
+
1002
614154
alt.fact.
101
alternating
30
56
+
8349
10143
amenable
56
77
+
851376628
952050665
apocalyptic
1002
3010
+
21637
26015
arithmetic
11
15
+
8118264
9289091
astonishing
15
Bell
15
betrothed
1575
binomial
15
56
231
792
brilliant
15
2679689
c.decagonal
11
101
c.heptagonal
22
cake
15
42
176
canyon
101
627
8349
Catalan
42
Chen
11
101
congruent
15
22
+
7089500
8118264
constructible
15
30
Cullen
385
Cunningham
15
101
14883
Curzon
30
1958
+
614154
2679689
cyclic
11
15
+
6185689
9289091
D-number
15
d-powerful
135
1255
2679689
de Polignac
17977
21637
23338469
decagonal
297
deficient
11
15
+
6185689
9289091
dig.balanced
11
15
+
56634173
169229875
double fact.
15
Duffinian
77
385
+
6185689
9289091
eban
30
42
56
economical
11
15
+
147273
10619863
emirpimes
15
equidigital
11
15
+
147273
10619863
esthetic
56
101
4565
Eulerian
11
evil
15
30
+
851376628
952050665
Friedman
1255
gapful
135
176
+
12292341831
18440293320
Giuga
30
good prime
11
101
happy
176
490
+
4087968
7089500
Harshad
30
42
+
4351078600
4835271870
heptagonal
3010
hexagonal
15
231
hoax
22
627
+
7089500
8118264
iban
11
22
+
12310
147273
idoneal
15
22
+
42
385
inconsummate
26015
37338
+
526823
614154
interprime
15
30
+
15796476
20506255
Jacobsthal
11
junction
101
3718
+
831820
3087735
Kaprekar
297
katadrome
30
42
Lehmer
15
Lucas
11
lucky
15
135
+
4697205
5392783
Lynch-Bell
15
135
2436
magic
15
magnanimous
11
30
56
101
metadrome
15
56
135
modest
627
2436
Moran
42
mountain
176
231
+
12310
124754
nialpdrome
11
22
+
42
77
nude
11
15
+
2436
8118264
oban
11
15
+
385
627
octagonal
176
odious
11
22
+
607163746
679903203
palindromic
11
22
77
101
palprime
11
101
pancake
11
22
56
panconsummate
11
15
77
231
pandigital
11
15
135
239943
pentagonal
22
176
pernicious
11
22
+
6185689
9289091
Perrin
22
plaindrome
11
15
+
135
1255
practical
30
42
+
7089500
8118264
prim.abundant
30
42
+
614154
1741630
prime
11
101
+
80630964769
228204732751
primorial
30
productive
22
pronic
30
42
56
Proth
385
pseudoperfect
30
42
+
715220
831820
repdigit
11
22
77
repunit
15
Ruth-Aaron
15
77
self
42
176
+
431149389
541946240
self-describing
22
semiprime
15
22
+
72533807
82010177
sliding
11
101
Smith
22
627
1255
Sophie Germain
11
sphenic
30
42
+
23338469
64112359
straight-line
135
strobogrammatic
11
101
strong prime
11
101
17977
10619863
super Niven
30
1002
super-d
231
12310
+
4087968
7089500
tau
56
792
+
15796476
541946240
tetrahedral
56
tetranacci
15
56
triangular
15
231
twin
11
101
uban
11
15
+
56
77
Ulam
11
77
+
1505499
2012558
undulating
101
unprimeable
5604
12310
+
7089500
8118264
untouchable
792
1002
+
12310
831820
wasteful
22
30
+
8118264
9289091
Zuckerman
11
15
135
1575
Zumkeller
30
42
+
31185
37338
zygodrome
11
22
77