The first 600 admirable numbers :
12, 20, 24, 30, 40, 42, 54, 56, 66, 70, 78, 84, 88, 102, 104, 114, 120, 138, 140, 174, 186, 222, 224, 234, 246, 258, 270, 282, 308, 318, 354, 364, 366, 368, 402, 426, 438, 464, 474, 476, 498, 532, 534, 582, 606, 618, 642, 644, 650, 654, 672, 678, 762, 786, 812, 822, 834, 836, 868, 894, 906, 942, 945, 978, 992, 1002, 1036, 1038, 1074, 1086, 1146, 1148, 1158, 1182, 1194, 1204, 1266, 1316, 1338, 1362, 1372, 1374, 1398, 1434, 1446, 1484, 1488, 1504, 1506, 1542, 1578, 1614, 1626, 1638, 1652, 1662, 1686, 1698, 1708, 1758, 1842, 1866, 1876, 1878, 1888, 1902, 1952, 1986, 1988, 2002, 2022, 2044, 2082, 2094, 2118, 2154, 2202, 2212, 2238, 2274, 2298, 2324, 2334, 2382, 2406, 2454, 2480, 2492, 2514, 2526, 2586, 2598, 2634, 2658, 2694, 2716, 2742, 2766, 2778, 2802, 2828, 2874, 2884, 2922, 2946, 2994, 2996, 3018, 3052, 3054, 3126, 3138, 3164, 3230, 3246, 3250, 3282, 3342, 3378, 3414, 3426, 3462, 3472, 3522, 3556, 3558, 3594, 3606, 3642, 3668, 3678, 3702, 3714, 3724, 3770, 3786, 3836, 3846, 3858, 3882, 3892, 3918, 3954, 3966, 4030, 4038, 4062, 4095, 4098, 4146, 4172, 4206, 4228, 4254, 4314, 4362, 4396, 4398, 4434, 4458, 4506, 4542, 4564, 4566, 4614, 4638, 4676, 4722, 4730, 4782, 4844, 4854, 4866, 4926, 4938, 4962, 4974, 5012, 5034, 5068, 5118, 5142, 5154, 5178, 5262, 5286, 5298, 5322, 5348, 5404, 5442, 5456, 5466, 5514, 5516, 5572, 5574, 5622, 5624, 5646, 5682, 5718, 5802, 5826, 5830, 5862, 5898, 5908, 5946, 5982, 6054, 6078, 6114, 6126, 6186, 6198, 6200, 6234, 6244, 6294, 6306, 6356, 6366, 6378, 6412, 6414, 6435, 6448, 6522, 6524, 6546, 6558, 6582, 6618, 6654, 6692, 6702, 6738, 6748, 6774, 6906, 6918, 6978, 7026, 7028, 7086, 7122, 7158, 7192, 7196, 7206, 7278, 7302, 7338, 7364, 7374, 7386, 7422, 7425, 7494, 7532, 7554, 7588, 7662, 7674, 7698, 7734, 7746, 7756, 7782, 7806, 7818, 7842, 7868, 7912, 7914, 7924, 7926, 7962, 8166, 8202, 8204, 8238, 8286, 8394, 8415, 8432, 8454, 8538, 8562, 8574, 8596, 8598, 8634, 8682, 8706, 8708, 8718, 8754, 8764, 8826, 8876, 8886, 8898, 8922, 8925, 8934, 8958, 8994, 9066, 9112, 9138, 9186, 9258, 9268, 9294, 9318, 9354, 9402, 9424, 9426, 9436, 9474, 9498, 9555, 9582, 9606, 9642, 9654, 9678, 9714, 9716, 9726, 9762, 9772, 9822, 9884, 9942, 9978, 10002, 10014, 10052, 10158, 10182, 10194, 10254, 10276, 10326, 10338, 10398, 10444, 10446, 10482, 10518, 10554, 10612, 10662, 10698, 10722, 10724, 10734, 10792, 10806, 10866, 10892, 10938, 10986, 11082, 11096, 11116, 11166, 11202, 11226, 11228, 11238, 11262, 11274, 11334, 11406, 11408, 11442, 11452, 11478, 11586, 11598, 11694, 11706, 11732, 11788, 11838, 11874, 11922, 11958, 11982, 11994, 12018, 12066, 12068, 12102, 12124, 12162, 12174, 12234, 12292, 12318, 12378, 12404, 12414, 12486, 12498, 12522, 12534, 12572, 12594, 12666, 12678, 12774, 12786, 12796, 12822, 12846, 12858, 12908, 12918, 12964, 12966, 13074, 13076, 13218, 13242, 13278, 13326, 13412, 13422, 13434, 13458, 13506, 13602, 13614, 13636, 13638, 13686, 13722, 13736, 13748, 13758, 13782, 13854, 13866, 13972, 13998, 14034, 14046, 14082, 14084, 14106, 14142, 14226, 14252, 14262, 14286, 14298, 14334, 14358, 14384, 14394, 14466, 14502, 14538, 14588, 14622, 14644, 14646, 14682, 14754, 14802, 14838, 14862, 15018, 15126, 15148, 15186, 15234, 15258, 15294, 15306, 15316, 15342, 15474, 15546, 15558, 15596, 15654, 15702, 15726, 15764, 15798, 15872, 15882, 15932, 15942, 15954, 15978, 15988, 16026, 16062, 16098, 16122, 16134, 16156, 16158, 16194, 16242, 16256, 16266, 16278, 16314, 16374, 16386, 16436, 16446, 16494, 16518, 16602, 16604, 16662, 16734, 16746, 16772, 16782, 16806, 16818, 16828, 16914, 16996, 16998, 17022, 17058, 17106, 17142, 17164, 17166, 17272, 17274, 17276, 17322, 17332, 17382, 17418, 17454, 17502, 17562, 17634, 17668, 17718, 17742, 17778, 17814, 17816, 17826.
Distribution of the remainders when the numbers in this family are divided by n=2, 3,..., 11. (I took into account 1345934 values, from 12 to 99999956).
| n\r | 0 | 1 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 1345818 | 116 | 2 | ||||||||
| 3 | 1071336 | 137208 | 137390 | 3 | |||||||
| 4 | 274563 | 55 | 1071255 | 61 | 4 | ||||||
| 5 | 145 | 336332 | 336371 | 336561 | 336525 | 5 | |||||
| 6 | 1071220 | 0 | 137390 | 116 | 137208 | 0 | 6 | ||||
| 7 | 254925 | 181793 | 181928 | 181759 | 181927 | 181822 | 181780 | 7 | |||
| 8 | 19689 | 27 | 535773 | 27 | 254874 | 28 | 535482 | 34 | 8 | ||
| 9 | 104 | 45715 | 45775 | 535789 | 45755 | 45795 | 535443 | 45738 | 45820 | 9 | |
| 10 | 48 | 7 | 336369 | 4 | 336519 | 97 | 336325 | 2 | 336557 | 6 | 10 |
| 11 | 76 | 134627 | 134593 | 134724 | 134488 | 134603 | 134480 | 134583 | 134576 | 134586 | 134598 |
A pictorial representation of the table above
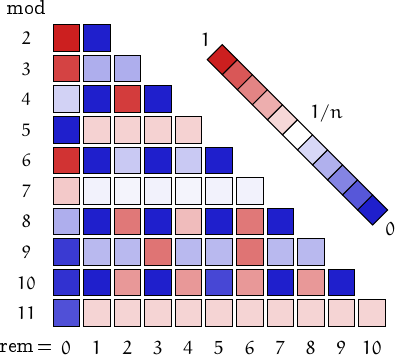
Imagine to divide the members of this family by a number n and compute the remainders. Should they be uniformly distributed, each remainder from 0 to n-1 would be obtained in about (1/n)-th of the cases. This outcome is represented by a white square. Reddish (resp. bluish) squares represent remainders which appear more (resp. less) frequently than 1/n.
e-mail: info -at- numbersaplenty.com • Privacy notice • engine limits