The first 600 d-powerful numbers :
1, 2, 3, 4, 5, 6, 7, 8, 9, 24, 43, 63, 89, 132, 135, 153, 175, 209, 224, 226, 262, 264, 267, 283, 332, 333, 334, 357, 370, 371, 372, 373, 374, 375, 376, 377, 378, 379, 407, 445, 463, 518, 598, 629, 739, 794, 849, 935, 994, 1034, 1073, 1074, 1234, 1255, 1306, 1323, 1326, 1349, 1364, 1386, 1498, 1542, 1634, 1672, 1676, 1765, 1836, 2048, 2062, 2063, 2064, 2083, 2114, 2130, 2131, 2132, 2133, 2134, 2135, 2136, 2137, 2138, 2139, 2179, 2194, 2203, 2205, 2223, 2224, 2225, 2227, 2240, 2241, 2242, 2243, 2244, 2245, 2246, 2247, 2248, 2249, 2263, 2264, 2283, 2314, 2315, 2332, 2333, 2334, 2335, 2336, 2352, 2353, 2355, 2356, 2372, 2373, 2376, 2379, 2394, 2395, 2396, 2407, 2423, 2427, 2443, 2445, 2449, 2464, 2465, 2483, 2517, 2532, 2536, 2537, 2554, 2572, 2574, 2577, 2597, 2607, 2623, 2626, 2627, 2628, 2645, 2664, 2733, 2735, 2737, 2738, 2739, 2753, 2757, 2773, 2792, 2793, 2794, 2803, 2824, 2843, 2849, 2863, 2864, 2932, 2933, 2934, 2939, 2953, 2973, 2994, 3145, 3147, 3165, 3167, 3212, 3214, 3215, 3234, 3235, 3236, 3237, 3238, 3252, 3254, 3255, 3257, 3274, 3292, 3294, 3295, 3298, 3324, 3342, 3345, 3362, 3385, 3432, 3435, 3436, 3437, 3453, 3454, 3457, 3474, 3475, 3476, 3477, 3492, 3496, 3523, 3525, 3527, 3543, 3566, 3569, 3612, 3635, 3652, 3657, 3674, 3678, 3692, 3706, 3723, 3725, 3726, 3746, 3749, 3764, 3765, 3786, 3856, 3940, 3941, 3942, 3943, 3944, 3945, 3946, 3947, 3948, 3949, 4114, 4132, 4133, 4136, 4150, 4151, 4152, 4153, 4154, 4155, 4156, 4157, 4158, 4159, 4173, 4194, 4209, 4224, 4225, 4228, 4240, 4241, 4242, 4243, 4244, 4245, 4246, 4247, 4248, 4249, 4260, 4261, 4262, 4263, 4264, 4265, 4266, 4267, 4268, 4269, 4285, 4288, 4316, 4332, 4339, 4352, 4353, 4355, 4373, 4396, 4397, 4403, 4405, 4424, 4443, 4447, 4449, 4469, 4485, 4572, 4573, 4574, 4599, 4622, 4623, 4624, 4644, 4648, 4649, 4685, 4732, 4733, 4735, 4736, 4738, 4826, 4849, 4869, 4932, 4933, 4950, 4951, 4952, 4953, 4954, 4955, 4956, 4957, 4958, 4959, 5182, 5232, 5236, 5258, 5259, 5276, 5320, 5321, 5322, 5323, 5324, 5325, 5326, 5327, 5328, 5329, 5342, 5343, 5344, 5364, 5366, 5367, 5384, 5432, 5453, 5547, 5562, 5567, 5632, 5653, 5657, 5746, 5766, 5832, 5833, 5858, 5873, 5877, 5902, 6244, 6247, 6249, 6423, 6424, 6443, 6579, 6603, 6649, 6669, 6714, 6732, 6792, 6793, 6794, 6849, 6932, 6934, 6935, 6937, 6939, 6972, 6974, 6976, 7032, 7123, 7234, 7279, 7296, 7299, 7323, 7329, 7343, 7363, 7432, 7582, 7632, 7639, 7836, 7906, 7928, 7944, 7962, 7964, 8200, 8201, 8202, 8203, 8204, 8205, 8206, 8207, 8208, 8209, 8223, 8224, 8225, 8228, 8243, 8245, 8262, 8263, 8283, 8288, 8289, 8316, 8352, 8376, 8396, 8443, 8462, 8536, 8623, 8647, 8667, 8712, 8756, 8792, 8825, 8864, 8932, 8970, 8971, 8972, 8973, 8974, 8975, 8976, 8977, 8978, 8979, 9122, 9234, 9236, 9237, 9272, 9273, 9274, 9324, 9342, 9347, 9362, 9385, 9474, 9478, 9493, 9654, 9693, 9697, 9723, 9725, 9947, 9963, 10322, 10693, 10694, 10722, 10723, 12298, 12324, 12343, 12345, 12347, 12367, 12415, 12432, 12434, 12495, 12542, 12562, 12615, 12634, 12695, 12723, 12854, 13132, 13133, 13173, 13332, 13337, 13373, 13393, 13443, 13532, 13933, 13978, 14276, 14295, 14346, 14362, 14363, 14369, 14697, 14722, 14726, 14786, 15422, 15423, 15686, 15822, 15882, 16213, 16234, 16274, 16293, 16346, 16363, 16430, 16431, 16432, 16433, 16434, 16435, 16436, 16437, 16438, 16439, 16453, 16470, 16471, 16472, 16473, 16474, 16475, 16476, 16477, 16478, 16479, 16494, 16524, 16543, 16634, 16729, 16742, 16743, 16857, 16927, 16942, 16984, 17026, 17133, 17157, 17224, 17240, 17241, 17242, 17243, 17244, 17245, 17246, 17247, 17248, 17249, 17287, 17332, 17334, 17338, 17352, 17357, 17372, 17376, 17378, 17407, 17424, 17443, 17445, 17448, 17463.
Distribution of the remainders when the numbers in this family are divided by n=2, 3,..., 11. (I took into account 374482 values, from 1 to 9999865).
| n\r | 0 | 1 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 189378 | 185104 | 2 | ||||||||
| 3 | 125018 | 124479 | 124985 | 3 | |||||||
| 4 | 91773 | 90882 | 97605 | 94222 | 4 | ||||||
| 5 | 49506 | 52972 | 101288 | 88141 | 82575 | 5 | |||||
| 6 | 63379 | 61615 | 63135 | 61639 | 62864 | 61850 | 6 | ||||
| 7 | 53759 | 53403 | 53449 | 53254 | 53408 | 53500 | 53709 | 7 | |||
| 8 | 45273 | 45133 | 51557 | 48648 | 46500 | 45749 | 46048 | 45574 | 8 | ||
| 9 | 41217 | 42211 | 40346 | 42594 | 40669 | 42422 | 41207 | 41599 | 42217 | 9 | |
| 10 | 8972 | 8973 | 58278 | 56847 | 46835 | 40534 | 43999 | 43010 | 31294 | 35740 | 10 |
| 11 | 35929 | 32173 | 35531 | 33205 | 34580 | 34137 | 33389 | 35214 | 32379 | 35728 | 32217 |
A pictorial representation of the table above
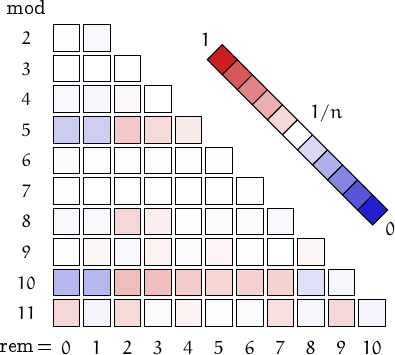
Imagine to divide the members of this family by a number n and compute the remainders. Should they be uniformly distributed, each remainder from 0 to n-1 would be obtained in about (1/n)-th of the cases. This outcome is represented by a white square. Reddish (resp. bluish) squares represent remainders which appear more (resp. less) frequently than 1/n.
e-mail: info -at- numbersaplenty.com • Privacy notice • engine limits