For example,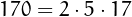 
and 
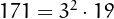form a pair since
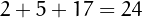and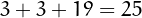 
.
The pairs below 10000 are (2, 3), (3, 4), (4, 5), (9, 10), (20, 21), (24, 25), (98, 99), (170, 171), (1104, 1105), (1274, 1275), (2079, 2080), (2255, 2256), (3438, 3439), (4233, 4234), (4345, 4346), (4716, 4717), (5368, 5369), (7105, 7106), and (7625, 7626). more terms
Up to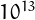 
there are only 5 eRat triples, namely
(2, 3, 4), (3, 4, 5), (27574665988, 27574665989, 27574665990),
(1862179264458, 1862179264459, 1862179264460), and
(9600314395008, 9600314395009, 9600314395010).
For the smallest nontrivial triple we have
![\[
\begin{array}{lll}
27574665988 &=& 2^2 \cdot 139 \cdot 269 ⋅\cdot 331 \cdot 557\\
27574665989 &=& 13 \cdot 41 \cdot 191 \cdot 439 \cdot 617\\
27574665990 &=& 2 \cdot 3 \cdot 5 \cdot 19 \cdot 163 \cdot 449 \cdot 661\,,
\end{array}
\]](pic.6.png)
Devesh defines the "depth of an eRAP"
as the number of levels through which this property holds true.
For example, the pair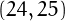 
is of depth 2, because applying the function
sum of prime factors we have 
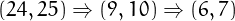and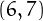 
is not an eRAP.
Up to 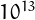there are 9 eRAPs of depth 5. The smallest one is
![\[\small
\left(\begin{array}{c}2957791666084\\2957791666085\end{array}\right)\!\Rightarrow\!
\left(\begin{array}{c}121539\\121540\end{array}\right)\!\Rightarrow\!
\left(\begin{array}{c}170\\171\end{array}\right)\!\Rightarrow\!
\left(\begin{array}{c}24\\25\end{array}\right)\!\Rightarrow\!
\left(\begin{array}{c}9\\10\end{array}\right)\!\Rightarrow\!
\left(\begin{array}{c}6\\7\end{array}\right).
\]](pic.10.png)
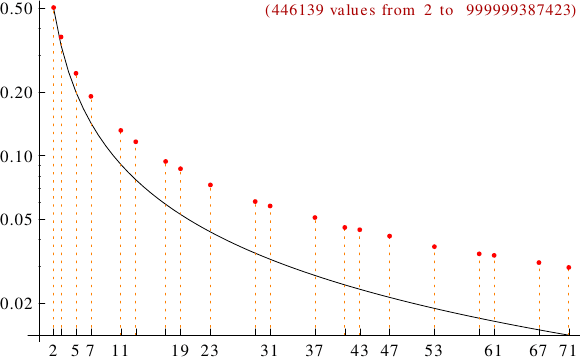
You can download a text file (eRAP_upto1e12.txt) of 5.4 MB, containing the first members of the
446139
eRAPs up to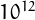 
.
