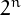 is called apocalyptic if its digits contain "666" as a substring.
is called apocalyptic if its digits contain "666" as a substring.
The smallest apocalyptic number is 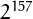 , which is equal to
, which is equal to
![\[
182687704\underline{666}362864775460604089535377456991567872\,,
\]](pic.3.png)
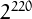 is the smallest apocalyptic number which contains two 666 groups, being
equal to
is the smallest apocalyptic number which contains two 666 groups, being
equal to168499666669691498716668844
The smallest power of 2 with 3 groups is
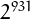 .
.
A number 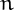 such that
such that 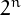 is apocalyptic is called apocalyptic power or apocalyptic exponent.
is apocalyptic is called apocalyptic power or apocalyptic exponent.
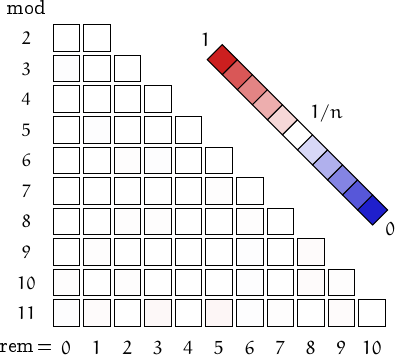
Between 1 and 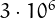 there are 3715 numbers which are non-apocalyptic exponents, the largest being 29784. In other words, it is highly probable that
there are 3715 numbers which are non-apocalyptic exponents, the largest being 29784. In other words, it is highly probable that 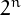 for
for 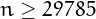 is an apocalyptic number.
is an apocalyptic number.
Probably there are only 8 numbers, namely 2666, 3666, 5666, 6660, 6665, 6669, 11666, 26667 which contains 666 among their digits but are not apocalyptic exponents.
The first apocalyptic exponents are 157, 192, 218, 220, 222, 224, 226, 243, 245, 247, 251, 278, 285, 286, 287, 312, 355, 361, 366, 382, 384, 390, 394, 411, 434, 443, 478, 497, 499, 506 more terms
The smallest 3 × 3 magic square made of consecutive apocalyptic numbers is
| 1490 | 1478 | 1487 |
| 1482 | 1485 | 1488 |
| 1483 | 1492 | 1480 |
Below, the spiral pattern of apocalyptic numbers up to 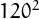 . See the page on prime numbers for an explanation and links to similar pictures.
. See the page on prime numbers for an explanation and links to similar pictures.