The first 600 iban numbers :
1, 2, 3, 4, 7, 10, 11, 12, 14, 17, 20, 21, 22, 23, 24, 27, 40, 41, 42, 43, 44, 47, 70, 71, 72, 73, 74, 77, 100, 101, 102, 103, 104, 107, 110, 111, 112, 114, 117, 120, 121, 122, 123, 124, 127, 140, 141, 142, 143, 144, 147, 170, 171, 172, 173, 174, 177, 200, 201, 202, 203, 204, 207, 210, 211, 212, 214, 217, 220, 221, 222, 223, 224, 227, 240, 241, 242, 243, 244, 247, 270, 271, 272, 273, 274, 277, 300, 301, 302, 303, 304, 307, 310, 311, 312, 314, 317, 320, 321, 322, 323, 324, 327, 340, 341, 342, 343, 344, 347, 370, 371, 372, 373, 374, 377, 400, 401, 402, 403, 404, 407, 410, 411, 412, 414, 417, 420, 421, 422, 423, 424, 427, 440, 441, 442, 443, 444, 447, 470, 471, 472, 473, 474, 477, 700, 701, 702, 703, 704, 707, 710, 711, 712, 714, 717, 720, 721, 722, 723, 724, 727, 740, 741, 742, 743, 744, 747, 770, 771, 772, 773, 774, 777, 1000, 1001, 1002, 1003, 1004, 1007, 1010, 1011, 1012, 1014, 1017, 1020, 1021, 1022, 1023, 1024, 1027, 1040, 1041, 1042, 1043, 1044, 1047, 1070, 1071, 1072, 1073, 1074, 1077, 1100, 1101, 1102, 1103, 1104, 1107, 1110, 1111, 1112, 1114, 1117, 1120, 1121, 1122, 1123, 1124, 1127, 1140, 1141, 1142, 1143, 1144, 1147, 1170, 1171, 1172, 1173, 1174, 1177, 1200, 1201, 1202, 1203, 1204, 1207, 1210, 1211, 1212, 1214, 1217, 1220, 1221, 1222, 1223, 1224, 1227, 1240, 1241, 1242, 1243, 1244, 1247, 1270, 1271, 1272, 1273, 1274, 1277, 1300, 1301, 1302, 1303, 1304, 1307, 1310, 1311, 1312, 1314, 1317, 1320, 1321, 1322, 1323, 1324, 1327, 1340, 1341, 1342, 1343, 1344, 1347, 1370, 1371, 1372, 1373, 1374, 1377, 1400, 1401, 1402, 1403, 1404, 1407, 1410, 1411, 1412, 1414, 1417, 1420, 1421, 1422, 1423, 1424, 1427, 1440, 1441, 1442, 1443, 1444, 1447, 1470, 1471, 1472, 1473, 1474, 1477, 1700, 1701, 1702, 1703, 1704, 1707, 1710, 1711, 1712, 1714, 1717, 1720, 1721, 1722, 1723, 1724, 1727, 1740, 1741, 1742, 1743, 1744, 1747, 1770, 1771, 1772, 1773, 1774, 1777, 2000, 2001, 2002, 2003, 2004, 2007, 2010, 2011, 2012, 2014, 2017, 2020, 2021, 2022, 2023, 2024, 2027, 2040, 2041, 2042, 2043, 2044, 2047, 2070, 2071, 2072, 2073, 2074, 2077, 2100, 2101, 2102, 2103, 2104, 2107, 2110, 2111, 2112, 2114, 2117, 2120, 2121, 2122, 2123, 2124, 2127, 2140, 2141, 2142, 2143, 2144, 2147, 2170, 2171, 2172, 2173, 2174, 2177, 2200, 2201, 2202, 2203, 2204, 2207, 2210, 2211, 2212, 2214, 2217, 2220, 2221, 2222, 2223, 2224, 2227, 2240, 2241, 2242, 2243, 2244, 2247, 2270, 2271, 2272, 2273, 2274, 2277, 2300, 2301, 2302, 2303, 2304, 2307, 2310, 2311, 2312, 2314, 2317, 2320, 2321, 2322, 2323, 2324, 2327, 2340, 2341, 2342, 2343, 2344, 2347, 2370, 2371, 2372, 2373, 2374, 2377, 2400, 2401, 2402, 2403, 2404, 2407, 2410, 2411, 2412, 2414, 2417, 2420, 2421, 2422, 2423, 2424, 2427, 2440, 2441, 2442, 2443, 2444, 2447, 2470, 2471, 2472, 2473, 2474, 2477, 2700, 2701, 2702, 2703, 2704, 2707, 2710, 2711, 2712, 2714, 2717, 2720, 2721, 2722, 2723, 2724, 2727, 2740, 2741, 2742, 2743, 2744, 2747, 2770, 2771, 2772, 2773, 2774, 2777, 3000, 3001, 3002, 3003, 3004, 3007, 3010, 3011, 3012, 3014, 3017, 3020, 3021, 3022, 3023, 3024, 3027, 3040, 3041, 3042, 3043, 3044, 3047, 3070, 3071, 3072, 3073, 3074, 3077, 3100, 3101, 3102, 3103, 3104, 3107, 3110, 3111, 3112, 3114, 3117, 3120, 3121, 3122, 3123, 3124, 3127, 3140, 3141, 3142, 3143, 3144, 3147, 3170, 3171, 3172, 3173, 3174, 3177, 3200, 3201, 3202, 3203, 3204, 3207, 3210, 3211, 3212, 3214, 3217, 3220, 3221, 3222, 3223, 3224, 3227, 3240, 3241, 3242, 3243.
Distribution of the remainders when the numbers in this family are divided by n=2, 3,..., 11. (I took into account 30275 values, from 1 to 777777).
| n\r | 0 | 1 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 15659 | 14616 | 2 | ||||||||
| 3 | 10092 | 10069 | 10114 | 3 | |||||||
| 4 | 8351 | 6264 | 7308 | 8352 | 4 | ||||||
| 5 | 5219 | 5220 | 10440 | 4176 | 5220 | 5 | |||||
| 6 | 5219 | 4849 | 5220 | 4873 | 5220 | 4894 | 6 | ||||
| 7 | 4365 | 4323 | 4321 | 4311 | 4311 | 4321 | 4323 | 7 | |||
| 8 | 4175 | 3132 | 3654 | 4176 | 4176 | 3132 | 3654 | 4176 | 8 | ||
| 9 | 3369 | 3357 | 3369 | 3360 | 3350 | 3370 | 3363 | 3362 | 3375 | 9 | |
| 10 | 5219 | 5220 | 5220 | 4176 | 5220 | 0 | 0 | 5220 | 0 | 0 | 10 |
| 11 | 2787 | 2766 | 2746 | 2752 | 2751 | 2729 | 2729 | 2751 | 2752 | 2746 | 2766 |
A pictorial representation of the table above
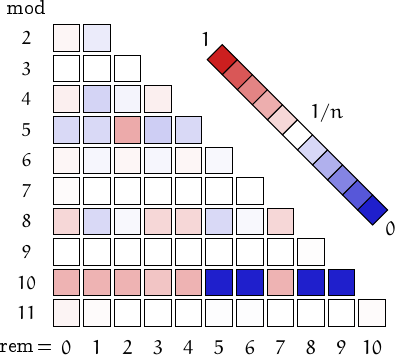
Imagine to divide the members of this family by a number n and compute the remainders. Should they be uniformly distributed, each remainder from 0 to n-1 would be obtained in about (1/n)-th of the cases. This outcome is represented by a white square. Reddish (resp. bluish) squares represent remainders which appear more (resp. less) frequently than 1/n.
e-mail: info -at- numbersaplenty.com • Privacy notice • engine limits